The data from the 62-cm experiment suggest an approximately linear relationship of seeing FWHM with the temperature delta for any given airflow speed. This reflects the fact that also in these conditions the seeing is caused by buoyant intermittent fluctuations like in the free convection case. Forced ventilation reduces this seeing by creating a mixed convection regime, which should be here characterized by the non-dimensional Froude number:
where D is the mirror diameter. We would therefore expect that the Froude number has the role of a stability parameter with respect to the FWHM normalized with the mirror-air temperature temperature difference. If this is the case the rate of mirror seeing with respect to the mirror-air temperature temperature difference is a function of the Froude number:
The results of both the 25-cm and 62-cm experiments are plotted against
the Froude number in fig.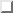 
. They do follow a function of
the Froude number as assumed by equation ()
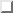which has the form:
Equations (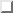) and (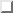) allow us to derive
an empirical similarity law for the scale 
of
mirror seeing from a ventilated mirror:
It follows that
The dependency on the Froude number and the
similarity relationship (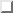) suggests a relationship
with the mirror size which would mean that larger mirrors
have more seeing in the same condition of ventilation, although this
is still to be verified on large mirrors.
